How to Find the Current in a Circuit

Chapter 5 Review

Key Terms

ampere (amp)
SI unit for current; $1~\mathrm{A}=1~\mathrm{C/s}$

circuit
complete path that an electrical current travels along

conventional current
current that flows through a circuit from the positive terminal of a battery through the circuit to the negative terminal of the battery

critical temperature
temperature at which a material reaches superconductivity

current density
flow of charge through a cross-sectional area divided by the area

diode
nonohmic circuit device that allows current flow in only one direction

drift velocity
velocity of a charge as it moves nearly randomly through a conductor, experiencing multiple collisions, averaged over a length of a conductor, whose magnitude is the length of conductor traveled divided by the time it takes for the charges to travel the length

electrical conductivity
measure of a material's ability to conduct or transmit electricity

electrical current
rate at which charge flows, $I=\frac{dQ}{dt}$

electrical power
time rate of change of energy in an electric circuit

Josephson junction
junction of two pieces of superconducting material separated by a thin layer of insulating material, which can carry a supercurrent

Meissner effect
phenomenon that occurs in a superconducting material where all magnetic fields are expelled

nonohmic
type of a material for which Ohm's law is not valid

ohm
( $\Omega$ ) unit of electrical resistance, $1~\Omega=1~\mathrm{V/A}$

ohmic
type of a material for which Ohm's law is valid, that is, the voltage drop across the device is equal to the current times the resistance

Ohm's law
empirical relation stating that the current is proportional to the potential difference ; it is often written as where is the resistance

resistance
electric property that impedes current; for ohmic materials, it is the ratio of voltage to current,

resistivity
intrinsic property of a material, independent of its shape or size, directly proportional to the resistance, denoted by $\rho$

schematic
graphical representation of a circuit using standardized symbols for components and solid lines for the wire connecting the components

SQUID
(Superconducting Quantum Interference Device) device that is a very sensitive magnetometer, used to measure extremely subtle magnetic fields

superconductivity
phenomenon that occurs in some materials where the resistance goes to exactly zero and all magnetic fields are expelled, which occurs dramatically at some low critical temperature ()

Summary

5.1 Electrical Current

5.2 Model of Conduction in Metals

The current through a conductor depends mainly on the motion of free electrons.
When an electrical field is applied to a conductor, the free electrons in a conductor do not move through a conductor at a constant speed and direction; instead, the motion is almost random due to collisions with atoms and other free electrons.
Even though the electrons move in a nearly random fashion, when an electrical field is applied to the conductor, the overall velocity of the electrons can be defined in terms of a drift velocity.
The current density is a vector quantity defined as the current through an infinitesimal area divided by the area.
The current can be found from the current density, $I=\iint_{\mathrm{area}}\vec{\mathbf{J}}\cdot d\vec{\mathbf{A}}.$
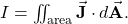
An incandescent light bulb is a filament of wire enclosed in a glass bulb that is partially evacuated. Current runs through the filament, where the electrical energy is converted to light and heat.

5.3 Resistivity and Resistance

5.4 Ohm's Law

5.5 Electrical Energy and Power

5.6 Superconductors

Superconductivity is a phenomenon that occurs in some materials when cooled to very low critical temperatures, resulting in a resistance of exactly zero and the expulsion of all magnetic fields.
Materials that are normally good conductors (such as copper, gold, and silver) do not experience superconductivity.
Superconductivity was first observed in mercury by Heike Kamerlingh Onnes in 1911. In 1986, Dr. Ching Wu Chu of Houston University fabricated a brittle, ceramic compound with a critical temperature close to the temperature of liquid nitrogen.
Superconductivity can be used in the manufacture of superconducting magnets for use in MRIs and high-speed, levitated trains.

Answers to Check Your Understanding

5.1 The time for $1.00~\mathrm{C}$ of charge to flow would be $\Delta t=\frac{\Delta Q}{I},$ slightly less than an hour. This is quite different from the $5.55~\mathrm{ms}$ for the truck battery. The calculator takes a very small amount of energy to operate, unlike the truck's starter motor. There are several reasons that vehicles use batteries and not solar cells. Aside from the obvious fact that a light source to run the solar cells for a car or truck is not always available, the large amount of current needed to start the engine cannot easily be supplied by present-day solar cells. Solar cells can possibly be used to charge the batteries. Charging the battery requires a small amount of energy when compared to the energy required to run the engine and the other accessories such as the heater and air conditioner. Present day solar-powered cars are powered by solar panels, which may power an electric motor, instead of an internal combustion engine.

5.2 The total current needed by all the appliances in the living room (a few lamps, a television, and your laptop) draw less current and require less power than the refrigerator.

5.3 The diameter of the -gauge wire is smaller than the diameter of the -gauge wire. Since the drift velocity is inversely proportional to the cross-sectional area, the drift velocity in the -gauge wire is larger than the drift velocity in the -gauge wire carrying the same current. The number of electrons per cubic meter will remain constant.

5.4 The current density in a conducting wire increases due to an increase in current. The drift velocity is inversely proportional to the current $\left(v_d=\frac{I}{nqA}\right),$ so the drift velocity would decrease.

5.5 Silver, gold, and aluminum are all used for making wires. All four materials have a high conductivity, silver having the highest. All four can easily be drawn into wires and have a high tensile strength, though not as high as copper. The obvious disadvantage of gold and silver is the cost, but silver and gold wires are used for special applications, such as speaker wires. Gold does not oxidize, making better connections between components. Aluminum wires do have their drawbacks. Aluminum has a higher resistivity than copper, so a larger diameter is needed to match the resistance per length of copper wires, but aluminum is cheaper than copper, so this is not a major drawback. Aluminum wires do not have as high of a ductility and tensile strength as copper, but the ductility and tensile strength is within acceptable levels. There are a few concerns that must be addressed in using aluminum and care must be used when making connections. Aluminum has a higher rate of thermal expansion than copper, which can lead to loose connections and a possible fire hazard. The oxidation of aluminum does not conduct and can cause problems. Special techniques must be used when using aluminum wires and components, such as electrical outlets, must be designed to accept aluminum wires.

5.6 The foil pattern stretches as the backing stretches, and the foil tracks become longer and thinner. Since the resistance is calculated as $R=\rho\frac{L}{A},$ the resistance increases as the foil tracks are stretched. When the temperature changes, so does the resistivity of the foil tracks, changing the resistance. One way to combat this is to use two strain gauges, one used as a reference and the other used to measure the strain. The two strain gauges are kept at a constant temperature

5.7 The longer the length, the smaller the resistance. The greater the resistivity, the higher the resistance. The larger the difference between the outer radius and the inner radius, that is, the greater the ratio between the two, the greater the resistance. If you are attempting to maximize the resistance, the choice of the values for these variables will depend on the application. For example, if the cable must be flexible, the choice of materials may be limited.

5.8 Yes, Ohm's law is still valid. At every point in time the current is equal to so the current is also a function of time, $I(t)=\frac{V_{\mathrm{max}}}{R}\sin(2\pi ft).$

5.9 Even though electric motors are highly efficient $10-20\%$ of the power consumed is wasted, not being used for doing useful work. Most of the $10-20\%$ of the power lost is transferred into heat dissipated by the copper wires used to make the coils of the motor. This heat adds to the heat of the environment and adds to the demand on power plants providing the power. The demand on the power plant can lead to increased greenhouse gases, particularly if the power plant uses coal or gas as fuel.

5.10 No, the efficiency is a very important consideration of the light bulbs, but there are many other considerations. As mentioned above, the cost of the bulbs and the life span of the bulbs are important considerations. For example, CFL bulbs contain mercury, a neurotoxin, and must be disposed of as hazardous waste. When replacing incandescent bulbs that are being controlled by a dimmer switch with LED, the dimmer switch may need to be replaced. The dimmer switches for LED lights are comparably priced to the incandescent light switches, but this is an initial cost which should be considered. The spectrum of light should also be considered, but there is a broad range of color temperatures available, so you should be able to find one that fits your needs. None of these considerations mentioned are meant to discourage the use of LED or CFL light bulbs, but they are considerations.

Conceptual Questions

5.1 Electrical Current

1. Can a wire carry a current and still be neutral—that is, have a total charge of zero? Explain.

2 . Car batteries are rated in ampere-hours ( $\mathrm{kW}\cdot\mathrm{h}$ ). To what physical quantity do ampere-hours correspond (voltage, current, charge, energy, power,…)?

3. When working with high-power electric circuits, it is advised that whenever possible, you work "one-handed" or "keep one hand in your pocket." Why is this a sensible suggestion?

5.2 Model of Conduction in Metals

4 . Incandescent light bulbs are being replaced with more efficient LED and CFL light bulbs. Is there any obvious evidence that incandescent light bulbs might not be that energy efficient? Is energy converted into anything but visible light?

5. It was stated that the motion of an electron appears nearly random when an electrical field is applied to the conductor. What makes the motion nearly random and differentiates it from the random motion of molecules in a gas?

6 . Electric circuits are sometimes explained using a conceptual model of water flowing through a pipe. In this conceptual model, the voltage source is represented as a pump that pumps water through pipes and the pipes connect components in the circuit. Is a conceptual model of water flowing through a pipe an adequate representation of the circuit? How are electrons and wires similar to water molecules and pipes? How are they different?

7. An incandescent light bulb is partially evacuated. Why do you suppose that is?

5.3 Resistivity and Resistance

8 . The drop across a resistor means that there is a change in potential or voltage across the resistor. Is there any change in current as it passes through a resistor? Explain.

9. Do impurities in semiconducting materials listed in Table 5.3.1 supply free charges? (Hint: Examine the range of resistivity for each and determine whether the pure semiconductor has the higher or lower conductivity.)

10 . Does the resistance of an object depend on the path current takes through it? Consider, for example, a rectangular bar—is its resistance the same along its length as across its width?

Pictures are a schematic drawing of a resistance object with the long side of the length R and the short side of the length R prime. In the left picture, current flows along the long side; in the right picture, current flows along the short side.

11. If aluminum and copper wires of the same length have the same resistance, which has the larger diameter? Why?

5.4 Ohm's Law

12 . In Determining Field from Potential, resistance was defined $R\equiv\frac{V}{I}.$ In this section, we presented Ohm's law, which is commonly expressed as The equations look exactly alike. What is the difference between Ohm's law and the definition of resistance?

13. Shown below are the results of an experiment where four devices were connected across a variable voltage source. The voltage is increased and the current is measured. Which device, if any, is an ohmic device?

Figure is a plot of current versus voltage. For A, current originally increases with voltage, then saturates and remains the same. For B, current linearly increases with voltage. For C current increases with voltage at a growing late. For D current decreases with voltage approaching zero.

5.5 Electrical Energy and Power

15. Common household appliances are rated at $110~\mathrm{V},$ but power companies deliver voltage in the kilovolt range and then step the voltage down using transformers to $110~\mathrm{V}$ to be used in homes. You will learn in later chapters that transformers consist of many turns of wire, which warm up as current flows through them, wasting some of the energy that is given off as heat. This sounds inefficient. Why do the power companies transport electric power using this method?

16 . Your electric bill gives your consumption in units of kilowatt-hour ( $\mathrm{kW}\cdot\mathrm{h}$ ). Does this unit represent the amount of charge, current, voltage, power, or energy you buy?

18 . An immersion heater is a small appliance used to heat a cup of water for tea by passing current through a resistor. If the voltage applied to the appliance is doubled, will the time required to heat the water change? By how much? Is this a good idea?

5.6 Superconductors

19. What requirement for superconductivity makes current superconducting devices expensive to operate?

20 . Name two applications for superconductivity listed in this section and explain how superconductivity is used in the application. Can you think of a use for superconductivity that is not listed?

Problems

5.1 Electrical Current

21. A Van de Graaff generator is one of the original particle accelerators and can be used to accelerate charged particles like protons or electrons. You may have seen it used to make human hair stand on end or produce large sparks. One application of the Van de Graaff generator is to create X-rays by bombarding a hard metal target with the beam. Consider a beam of protons at $1.00~\mathrm{keV}$ and a current of $5.00~\mathrm{mA}$ produced by the generator. (a) What is the speed of the protons? (b) How many protons are produced each second?

22 . A cathode ray tube (CRT) is a device that produces a focused beam of electrons in a vacuum. The electrons strike a phosphor-coated glass screen at the end of the tube, which produces a bright spot of light. The position of the bright spot of light on the screen can be adjusted by deflecting the electrons with electrical fields, magnetic fields, or both. Although the CRT tube was once commonly found in televisions, computer displays, and oscilloscopes, newer appliances use a liquid crystal display (LCD) or plasma screen. You still may come across a CRT in your study of science. Consider a CRT with an electron beam average current of $25.00~\mu\mathrm{A}.$ How many electrons strike the screen every minute?

23. How many electrons flow through a point in a wire in $3.00~\mathrm{s}$ if there is a constant current of $I=4.00~\mathrm{A}$ ?

25. The quantity of charge through a conductor is modelled as $Q=(4.00~\mathrm{C/s}^4)t^4-(1.00~\mathrm{C/s})t+6.00~\mathrm{mC}.$ What is the current at time $t=3.00~\mathrm{s}$ ?

26 . The current through a conductor is modelled as $I(t)=I_m\sin[2\pi(60~\mathrm{Hz})t].$ Write an equation for the charge as a function of time.

27. The charge on a capacitor in a circuit is modelled as $Q(t)=Q_{\mathrm{max}}\cos(\omega t+\phi).$ What is the current through the circuit as a function of time?

5.2 Model of Conduction in Metals

29. The current of an electron beam has a measured current of $I=50.00~\mu\mathrm{A}$ with a radius of $1.00~\mathrm{mm}^2.$ What is the magnitude of the current density of the beam?

32 . The current of an electron beam has a measured current of $I=50.00~\mu\mathrm{A}$ with a radius of $1.00~\mathrm{mm}^2.$ What is the magnitude of the current density of the beam?

5.3 Resistivity and Resistance

34 . What current flows through the bulb of a $3.00{\text -}\mathrm{V}$ flashlight when its hot resistance is $3.60~\Omega$ ?

35. Calculate the effective resistance of a pocket calculator that has a $1.35{\text -}\mathrm{V}$ battery and through which $0.200~\mathrm{mA}$ flows.

36 . How many volts are supplied to operate an indicator light on a DVD player that has a resistance of $140~\Omega,$ given that $25.0~\mathrm{mA}$ passes through it?

40 . A lead rod has a length of $30.00~\mathrm{cm}$ and a resistance of $5.00~\mu\Omega.$ What is the radius of the rod?

41. Find the ratio of the diameter of aluminum to copper wire, if they have the same resistance per unit length (as they might in household wiring).

43. (a) To what temperature must you raise a copper wire, originally at $20.0~^{\circ}\mathrm{C},$ to double its resistance, neglecting any changes in dimensions? (b) Does this happen in household wiring under ordinary circumstances?

44 . A resistor made of nichrome wire is used in an application where its resistance cannot change more than $1.00\%$ from its value at $20.0~^{\circ}\mathrm{C}.$ Over what temperature range can it be used?

46 . An electronic device designed to operate at any temperature in the range from $-10.0~^{\circ}\mathrm{C}$ to $55.0~^{\circ}\mathrm{C}$ contains pure carbon resistors. By what factor does their resistance increase over this range?

48 . Assuming a constant temperature coefficient of resistivity, what is the maximum percent decrease in the resistance of a constantan wire starting at $20.0~^{\circ}\mathrm{C}$ ?

5.4 Ohm's Law

50 . A $2.2{\text -}\mathrm{k}\Omega$ resistor is connected across a D cell battery ( $1.5~\mathrm{V}$ ). What is the current through the resistor?

52 . A resistor is connected in series with a power supply of $20.00~\mathrm{V}.$ The current measure is $0.50~\mathrm{A}.$ What is the resistance of the resistor?

53. A resistor is placed in a circuit with an adjustable voltage source. The voltage across and the current through the resistor and the measurements are shown below. Estimate the resistance of the resistor.

Figure is a plot of voltage versus current. There is a linear relationship between voltage and the current. It is zero Volts at zero Amperes, 200 Volts at 2 Amperes, 400 Volts at 4 Amperes, 600 Volts at 6 Amperes, and 800 Volts at 8 Amperes.

5.5 Electrical Energy and Power

55. A $20.0{\text -}\mathrm{V}$ battery is used to supply current to a $10{\text -}\mathrm{k}\Omega$ resistor. Assume the voltage drop across any wires used for connections is negligible. (a) What is the current through the resistor? (b) What is the power dissipated by the resistor? (c) What is the power input from the battery, assuming all the electrical power is dissipated by the resistor? (d) What happens to the energy dissipated by the resistor?

56 . What is the maximum voltage that can be applied to a $10{\text -}\mathrm{k}\Omega$ resistor rated at $14~\mathrm{W}$ ?

59. The power dissipated by a resistor with a resistance of $R=100~\Omega$ is $P=2.0~\mathrm{W}.$ What are the current through and the voltage drop across the resistor?

62 . A $0.50{\text -}\mathrm{W},$ $220{\text -}\Omega$ resistor carries the maximum current possible without damaging the resistor. If the current were reduced to half the value, what would be the power consumed?

5.6 Superconductors

64 . A wire is drawn through a die, stretching it to four times its original length. By what factor does its resistance increase?

68 . What is the change in temperature required to decrease the resistance for a carbon resistor by $10\%$ ?

Additional Problems

71. A nichrome rod that is $3.00~\mathrm{mm}$ long with a cross-sectional area of $1.00~\mathrm{mm}^2$ is used for a digital thermometer. (a) What is the resistance at room temperature? (b) What is the resistance at body temperature?

72. The temperature in Philadelphia, PA can vary between $68.00~^{\circ}\mathrm{F}$ and $100.00~^{\circ}\mathrm{F}$ in one summer day. By what percentage will an aluminum wire's resistance change during the day?

74 . A wire with a resistance of $5.0~\Omega$ is drawn out through a die so that its new length is twice times its original length. Find the resistance of the longer wire. You may assume that the resistivity and density of the material are unchanged.

78 . A -ohm, -watt resistor is placed in series with a power supply. (a) What is the maximum voltage that can be applied to the resistor without harming the resistor? (b) What would be the current through the resistor?

79. A battery with an emf of $24.00~\mathrm{V}$ delivers a constant current of $2.00~\mathrm{mA}$ to an appliance. How much work does the battery do in three minutes?

80 . A $12.00{\text -}\mathrm{V}$ battery has an internal resistance of a tenth of an ohm. (a) What is the current if the battery terminals are momentarily shorted together? (b) What is the terminal voltage if the battery delivers amps to a circuit?

Challenge Problems

86 . Consider a square rod of material with sides of length $L=3.00~\mathrm{cm}$ with a current density of $\vec{\mathbf{J}}=J_0e^{\alpha x}\hat{\mathbf{k}}=(0.35~\mathrm{A/m}^2)e^{(2.1\times10^{-3}~\mathrm{m}^{-1})x}\hat{\mathbf{k}}$ as shown below. Find the current that passes through the face of the rod.

Picture shows a co-ordinate axis with the square rod placed over it. It has dimensions of L in j and i directions. Current is flowing into the k direction through the area dx.

How to Find the Current in a Circuit

Source: https://openpress.usask.ca/physics155/chapter/chapter-5-review/

Anton Conferverd57

How to Find the Current in a Circuit

Chapter 5 Review

Key Terms

Summary

5.1 Electrical Current

5.2 Model of Conduction in Metals

5.3 Resistivity and Resistance

5.4 Ohm's Law

5.5 Electrical Energy and Power

5.6 Superconductors

Answers to Check Your Understanding

Conceptual Questions

5.1 Electrical Current

5.2 Model of Conduction in Metals

5.3 Resistivity and Resistance

5.4 Ohm's Law

5.5 Electrical Energy and Power

5.6 Superconductors

Problems

5.1 Electrical Current

5.2 Model of Conduction in Metals

5.3 Resistivity and Resistance

5.4 Ohm's Law

5.5 Electrical Energy and Power

5.6 Superconductors

Additional Problems

Challenge Problems

Candela Citations

Menu Halaman Statis